Too hot, too cold, just right

by Burkard Polster and Marty Ross

The Age, 6 August 2007

Imagine Goldilocks goes to the cottage of the One Bear. She finds a single bowl of porridge, but it is too hot. Goldilocks decides to wait, but if she waits too long, the porridge will be too cold. However, at just the right moment, the porridge will be just right and she can eat “happily ever after”. (Well, as long as she eats quickly).

This simple idea is captured by the mathematical notion of continuity: since the temperature makes no dramatic jumps, we think of it as varying continuously. The Intermediate Value Theorem (IVT) then tells us that if the temperature begins higher than desired and ends up lower than desired then, at some intermediate time (though we don’t know when), the temperature will be just right.

IVT is very intuitive, but it is actually difficult to prove, and it has some surprising consequences. Returning to Goldilocks, for example, we find her at the Bear’s table, trying to eat her Just Right porridge. However, the floor of the cottage is uneven (bears are not great carpenters), and so the table is wobbling. As one often does, she looks for a magazine or a piece of paper to jam under one leg. Alas (bears are also not great readers), Goldilocks cannot find any paper. What is poor Goldilocks to do?

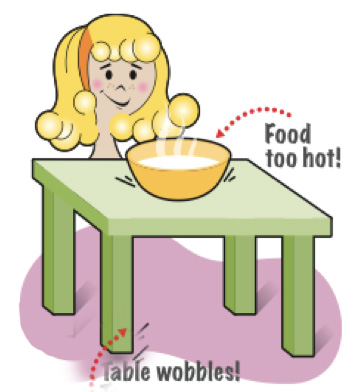
It is here that IVT comes to Goldilocks’ rescue. In fact, the table can be balanced - though perhaps still tilted - simply by rotating it on the spot. How can we see this?

To begin, balance three of the table legs on the ground, with the fourth leg hovering in the air. Then, try to rotate the table a quarter-turn, keeping the same three legs touching the ground. In fact - this is not so easy to see - the fourth leg will now be digging into the ground. So, the leg started up too high, and after rotating 90 degrees it ended up too low. So, by IVT, there is some intermediate angle where the fourth leg is just touching: the table is then balanced, and Goldilocks can again eat happily ever after.

(Disclaimer: The arguments above contain some technical assumptions and details, which we’ll explain to Goldilocks once she goes to University).