A puzzling Australia Day

by Burkard Polster and Marty Ross

The Age, 1 February 2010

Last Tuesday was Australia Day, and so a couple of patriotic Maths Masters met up to solve a 1000-piece jigsaw puzzle, a map of Australia. It was super-patriotic, since the map was covered with emus and echidnas and platypuses and the like.

We finished the puzzle, but only late in the night: our progress was slowed by the distracted pondering of jigsaw mathematics. We thought about the relationship between the number of pieces in a puzzle and the time to complete it. We wondered how the puzzlemakers ensure that each piece fits in just one spot in the puzzle. But, the question that really slowed us down was this: how simple can a 1000-piece jigsaw puzzle be?

An easy example is the tiling of a bathroom, consisting of identical squares: even blindfolded, anybody could complete such a "puzzle". The same would be true if our tiles were equilateral triangles or regular hexagons.

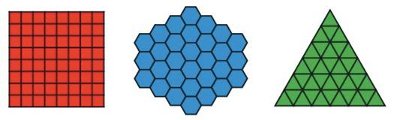
These three tilings with regular polygons are notable for their simplicity and symmetry, but no one is likely to be thrilled by a jigsaw puzzle consisting of 1000 red squares. There are other obvious choices of tile, such as rectangles and parallelograms, but they are hardly more interesting.

However, there are ingenious methods to transform simple tiles into complicated tiles in the form of real-life objects. These were pioneered by the brilliant graphic artist M.C.Escher. To view Escher's stunning, mathematically-inspired creations, visit the Symmetry Gallery at the M. C. Escher website.

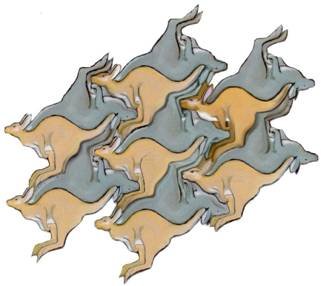
Oddly, Escher the Dutchman never used cuddly Australian animals in his work. This is where South Australian artist Bruce Bilney comes in. The kangaroo tiling above is his work, and below is another example: kangaroos interlaced with decorated Australias and possums. For many more example of Bilney's ingenious tilings, check out his Ozzigami website.

To see how Bilney creates his works, take a closer look at the tiling above. We have rotated it, and superimposed a square tiling. This demonstrates that Bilney's pattern can be regarded as a standard square tiling, but with each square decorated as shown on the right.

For the picture to tile properly, whatever sticks out from one side of the square has to match up with the other side. (Bilney has chosen to alternate red and grey kangaroos, which is why the colours don't match). But in fact that's all we have to worry about, and so we have an easy way to create interesting tilings: start with a square, cut out a couple of pieces from one edge, and reattach them on the opposite edge. The resulting shape can still be used as a tile.

By cutting out and reattaching more shapes, it is then easy to make complicated and striking tiles. Below, we have illustrated the steps involved in the making our own animal tile: the fabled coneheaded camel from the outback. Not a bad creation, from just a few cuts and pastes.

OK, so we admit it: our creation is not quite in the same league as those of Escher and Bilney. Think you can do better? Prove it! Email us your creations at mathsmasters@qedcat.com. We'll post the best ones on our website, and we'll offer a prize for our overall favourite.

Puzzle to Ponder: The rectangle superimposed on Bilney's masterpiece below is 16 centimeters wide and 13 centimeters high. What is the area of one of the kangaroos?

Feel free to use the comments section to suggest solutions. Later in the week we'll post our solutions on www.qedcat.com.

Burkard Polster teaches mathematics at Monash and is the university's resident mathemagician, mathematical juggler, origami expert, bubble-master, shoelace charmer, and Count von Count impersonator.

Marty Ross is a mathematical nomad. His hobby is smashing calculators with a hammer.

www.qedcat.com