Do prime ministers share their birthday cake?

by Burkard Polster and Marty Ross

The Age, 14 September 2009

Happy Birthday, Prime Minister Rudd! Well, almost. The PM's big day is September 21.

Did you know that our first prime minister, Edmund Barton, and our twenty-fourth prime minister, Paul Keating, were both born on January 18? And, did you know that the American presidents James Polk and Warren Harding share their birthday cake on January 2? Surely, these are amazing coincidences.

What are the chances that both among the 26 Australian prime ministers, and among the 44 American presidents, there are two sharing the same birthday? After all, there are 365 (and a quarter) possible birthdays, and very few leaders to compare. Or, maybe we just hunted for convenient data? What if we had instead checked the days on which our glorious leaders have died?

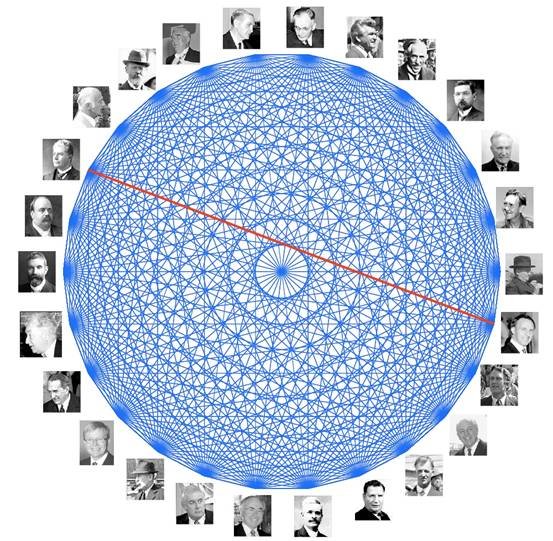
Probabilities can appear paradoxical. In fact, for any random group of 23 people, there is about a 50% chance that two of them share a birthday. This seems very surprising, but consider the diagram. When we are asking whether any two prime ministers have the same birthday, we are making as many comparisons as there are lines in the diagram – and there are a lot of lines! In reality, there are 325 lines hiding behind that innocent-looking 26.

Still don’t believe us? Okay, here is the maths. We shall sneak up on the problem: instead of calculating the chances of a shared birthday, we first calculate the “complementary” chance that there is no shared birthday. Whatever the birthday of Edmund Barton, the probability that the second prime minister has a different birthday must be 364/365.

Now that two birthdays are gone, the probability that the third prime minister has again a different birthday is 363/365. And so on. Then, the probability that no two of the 26 prime ministers share the same birthday is the product of all these probabilities:

So, there is a 40% chance that no two of our prime ministers have the same birthday. This leaves a 60% chance that two of them will share their cake.

The principle behind the birthday puzzle appears in many unexpected places. For example, imagine you rip 2000 songs onto your mp3 player, and switch it to shuffling songs randomly. If the player really chooses randomly from those 2000 songs, then there is a 50% chance that a song will be repeated among the first 53 chosen.

Now that we've shown you the secret of the birthday puzzle, here are your final exam questions. What are the chances that two of the 150 members of the Australian House of Representatives must share their birthday cake? And, what about the 435 members of the American House of Representatives?

www.qedcat.com