How to cook a telescope
Burkard Polster and Marty Ross
The Age, 13 October 2014

Home alone, taking care of the junior maths masters and it's dinner time. So, we'd better whip up a quick mathematical dish. Grab a pot, add some water and gelatine, give it all a quick spin. Voilà! An instant telescope.
Well, not exactly a telescope (and not much of a meal for those starving little maths masters). However some very pretty mathematics can be observed in this silly concoction.
Pour some water into a cylindrical bucket and spin the bucket around its axis. If the bucket has a constant rotational speed then the water will settle into a concave shape. But what shape?
Mathematikum, the wonderful mathematics museum in Giessen, Germany, has a very clever set-up to demonstrate what is happening. Instead of using a whole cylinder the museum begins with a narrow box, effectively a diametric slice of a cylinder.

The box sits on a turntable and when the turntable is spun around the red liquid inside climbs up the outer walls. The faster the box is spun, the higher the liquid climbs.

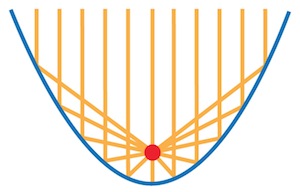
We can now see clearly the liquid formation and we can easily determine its shape. It's obviously not a straight line, and we remember from our school mathematics that not-straight things are always parabolas (those y = x2 things). So, it must be a parabola!
That's not a very convincing argument (we dearly hope), however the answer is indeed correct. We'll provide a more respectable and more mathematical argument below.
If the liquid in the box forms a parabola then spinning a full cylinder produces the surface obtained from rotating a parabola, what is known as a paraboloid. And that's where the telescope comes in.
Due to their well-known (if rarely proved) focal properties, parabolic mirrors (and lenses) are fundamental in the construction of telescopes. Traditionally such mirrors are constructed from grinding down a thick slab of glass. Unsurprisingly this is a very time-consuming and costly process, especially for the construction of large mirrors. Can this spinning trick be used to construct parabolic mirrors more easily and more cheaply? Indeed it can.
If we spin a bucket containing molten glass then the glass will cool into the shape of a paraboloid. (A hint for the newbies: don't use a plastic bucket.) The University of Arizona's mirror lab used the spinning trick to construct two parabolic mirrors over 8 meters in diameter, which are used in the aptly-named Large Binocular Telescope, pictured above. The mirrors for what will be largest telescope ever built are also being made with this technique.
While spinning mirrors is much less expensive than grinding them, the large mirrors still cost millions of dollars each to produce. However there is a related but much less expensive alternative.
Fill a large dish with mercury and spin it to create a paraboloid surface. This spinning surface can be used as mirror. The 6 meter mirror of the Large Zenith Telescope was constructed in this manner, for a fraction of the price of similarly sized solid mirrors. However the telescope does come with a significant drawback: it can only ever look straight up.
Now, back to work: why does the spinning liquid form a paraboloid? The solution probably dates back to the 17th century and Sir Isaac Newton. It is essentially a calculus problem, but the fundamental idea is not too difficult to explain.
Let's consider a little particle of mass m on the surface of the spinning water. The particle is a distance x from the axis of rotation and is spinning with some angular speed ω. Then the centrifugal "force" from the spinning must be balanced by gravitation and the outward push of the water:
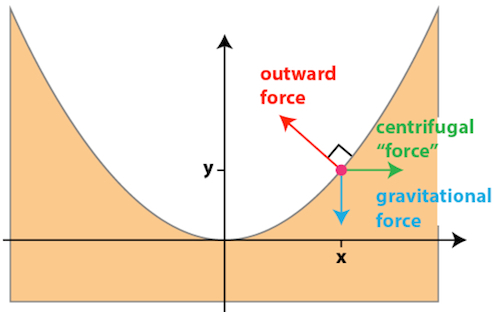
(For those who prefer to avoid use of the fictitious centrifugal force, the diagram above can be recast to show that the gravitational and outward forces must sum to give the non-fictitious centripetal force.)
But now the slope ("rise over run") of the liquid surface can be read off of the following diagram.
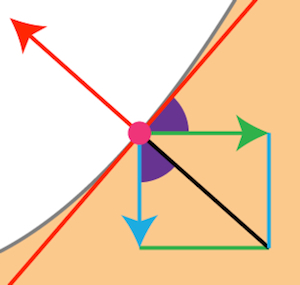
It follows that the slope equals (centrifugal force)/(gravitational force). With the well-known formula (mω2x) for the centrifugal force and with g standing for the gravitational acceleration, we then find
SLOPE = (ω2/g) x.
The calculus now kicks in, and it easily follows that the height y of the water particle is given by the equation
y = 1/2 (ω2/g) x2.
There's our parabola.
It is notable that the final equation only depends upon the angular speed and gravitational acceleration, and in particular not upon the material being spun. So, spinning mercury or water or Jello will all produce the same shape.
Which brings us back to our little maths masters. We put some Jello mix in a pot and spin it all on an old record turntable until the Jello sets. The result is a very impressive parabolic treat. Perhaps not the most nutritious dinner ever, but the little maths masters didn't appear to object.
Burkard Polster teaches mathematics at Monash and is the university's resident mathemagician, mathematical juggler, origami expert, bubble-master, shoelace charmer, and Count von Count impersonator.
Marty Ross is a mathematical nomad. His hobby is helping Barbie smash calculators and iPads with a hammer.
Copyright 2004-∞ ![]() All rights reserved.
All rights reserved.