The Nullarbor Conundrum

by Burkard Polster and Marty Ross

The Age, 3 August 2009

The Nullarbor Plain is one of Australia’s iconic geographical features, and taking the Indian-Pacific across the Nullarbor is one of the great train journeys. The trip includes the World’s longest straight section of railway line: 478 km without a bend.

The Nullarbor Plain is so flat and the railway line so straight, they are excellent models of the ideal planes and lines that mathematicians love. It was probably for this reason that the Nullarbor was chosen as the setting for this superb puzzle that first appeared in 1975 in the collection of puzzles Chez Angelique by J. Jaworski et al.:

Edward Eyre Junior is lost on the Nullarbor Plain. He hears a train whistle. Ed cannot see the train or the railway line, but he knows the direction from which the whistle came. His only chance of surviving is to run to a point on the line before the train gets to that point. In which direction should he run?

We can treat this as a purely mathematical puzzle, but let’s first consider a real-live Ed on the real Nullarbor. Then the railway line is basically running East-West. We’ll also assume Ed can read the sun or the stars well enough to know the direction of North. Then the scenario would be as pictured here:

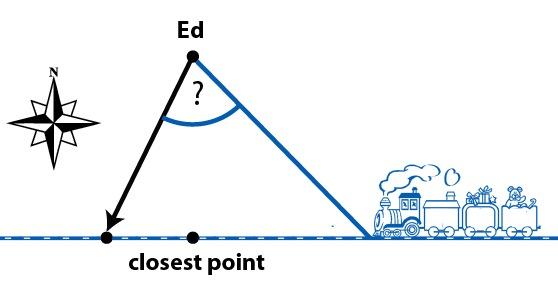
The closest point on the rail line is due South of Ed. Note that Ed may as well assume that the train is traveling towards this point. Otherwise, unless Ed can outrun the train, he is doomed no matter where he runs.

But this still leaves the question of the direction in which Ed should run. One would imagine that the correct choice depends upon the positions of Ed and the train, and of their speeds. Surprisingly, this is not the case.

Applying some simple trigonometry, it turns out that all the factors of distance and speed cancel: the very elegant solution is that Ed should always run at right angles to the original direction of the whistle. He may or may not be able to run fast enough, but if he can in fact save himself, that is the direction to go.

We won’t give the trigonometry details here, though if you recall the formulas it is very easy (all we need is the “sine rule”). You can find the details here. We also invite you to consider whether there is a direct geometric argument to determine Ed’s direction. Given that the answer is simply “go at right angles”, there may be such a simple argument. We’ll happily respond to any suggestions made in the comments below.

Finally, what if we consider the puzzle as purely mathematical, with Ideal Ed now lost on the Nullarbor PLANE? We’ll still assume the train is travelling along a straight line, but we no longer have compass directions to guide us. Does this change the puzzle? We’ll keep that for you to ponder. Please leave a comment below if you think you have the best advice for saving Ideal Ed.

www.qedcat.com