Choc-full of mathematics

Burkard Polster and Marty Ross

The Age, 24 February 2014

What can be more delightful than chocolate combined with mathematics? The answer, of course, is chocolate combined with more chocolate. Nonetheless, Austria's Mozartkugel is a very tasty mathematical treat.

The Mozartkugel is spherical, with layers of marzipan and nougat and the like, all coated in chocolate. The delicious specimen pictured above is from the Mirabell company but there are many manufacturers.

So which Mozartkugel is best? Ever willing to put in those long hours in the name of research, your Maths Masters conducted a comprehensive survey. Happily, it turns out they're all very good, but we've declared Mirabell the winner: as they love to boast, only Mirabell's Mozartkugel is truly spherical.

Having made our choice, and sampling one more Mozartkugel (just to make sure), we can begin to ponder the foil wrapper: how exactly does one wrap a spherical sweet? One way or another, it gets a little clumsy.

The Mirabell wrapper, pictured below, is a longish rectangle. To wrap the Mozartkugel we first form the wrapper into a cylinder, with the red line hugging the chocolate sphere along its equator. Then we just make it work, scrunching things together at the top and bottom.

Other manufacturers use square wrappers. In this case we begin with the north pole of the chocolate sphere touching the centre of the square, and then we scrunch things together at the south pole.

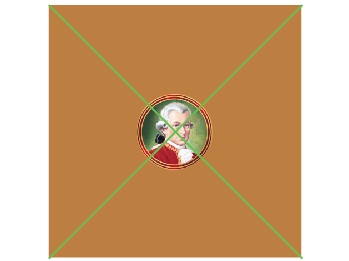
Now, an interesting question, especially for a manufacturer concerned to lower costs: which has less area, the rectangular wrapper or the square wrapper? It's a nice puzzle to ponder, so we've hidden the answer in the following paragraph.

The length of the rectangular wrapper must equal the circumference of the Mozartkugel, and its width must be half this circumference. So, if the Mozartkugel has a radius R then the area of the rectangular wrapper is 2πR x πR = 2π²R². (We're ignoring the little extra foil one would want to make sure there is some overlap.) Similarly, the diagonals of a square wrapper must also equal the circumference. With a little help from Pythagoras, it is then not difficult to show that the square wrapper also has an area of 2π²R²: exactly the same!

The perhaps surprising conclusion is that costwise it doesn't matter whether we use square wrappers or the longer rectangular wrappers. But we can do better than both.

Since rectangles fit together seamlessly there is no waste when cutting them from a large sheet of foil. Rather, the waste comes from the scrunching, and it turns out the square and rectangle have about 1.6 times the area of the sphere; about 36% of the foil gets scrunched. (We'll leave the true penny-pinchers to determine the precise figures.)

One alternative is to begin with a very long, very thin rectangle and wrap it around and around the sphere, as if pasting the peel back onto an apple. In this way we can lower the scrunch percentage to as close to 0 as desired. But of course such a wrapping would be entirely impractical, and it'd be pretty much impossible to include the pretty picture of Mozart. However, it turns out there are other, genuinely practical ways to significantly reduce scrunching.

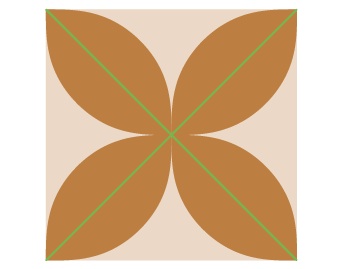
The simple and clever idea is to cut away chunks of foil that will only be scrunched anyway. For example, beginning with the Mirabell wrapper we can cut away curvy triangles, leaving four petals that will still wrap the sphere.(Renaissance cartographers used similar petal maps of the Earth in the construction of globes; for this purpose, small gaps were preferable to overlaps.)

However, it's a tricky question determining petal shapes that will work and are efficient. For example, choosing circular arcs for the edges will result in the petals being a little too skinny, leaving gaps in the wrapping. (We'll leave the proof of this as an interesting puzzle.)

Such petal wrappings were investigated in an elegant and fun (and technical) paper by mathematicians Erik Demaine, Martin Demaine, John Iacono and Stefan Langerman. They were inspired by Mozartkugel wrappings to determine the most efficient petal wrappings; in turn, their lovely paper inspired this column.

Demaine and his fellow petal masters determined the optimal petal shape to minimise scrunching. The final formula isn't so pretty, but the pretty petals are as pictured above. And, the scrunching percentage for this best petal wrapping is an impressively low 7%. (That can be further lowered by including more and thinner petals, but we would again lose our picture of Mozart.)

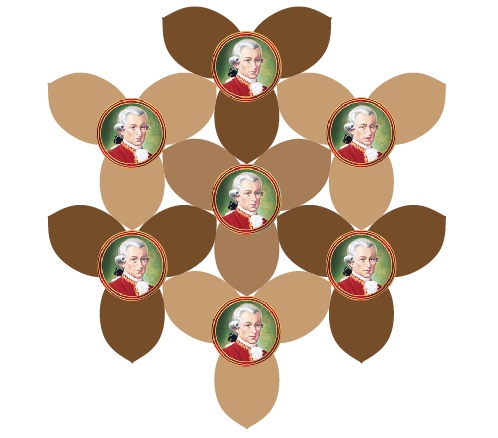
Of course, even if the scrunching percentage is low, we'll still be wasting (or at least be forced to recycle) the removed curvy triangles. However, the petal masters note that the petal wrappers can actually be very tightly packed, resulting in very little discarded foil:

And, we can make everything much neater by expanding each wrapper into a slightly longish hexagon, as pictured below. Now we just have to cut in straight lines, no foil is discarded and the scrunching percentage is still a respectably low 13%.

There are tons of other interesting material in the petal masters' paper. For example, they also observe that the same four petals can be used to replace the square wrapper:

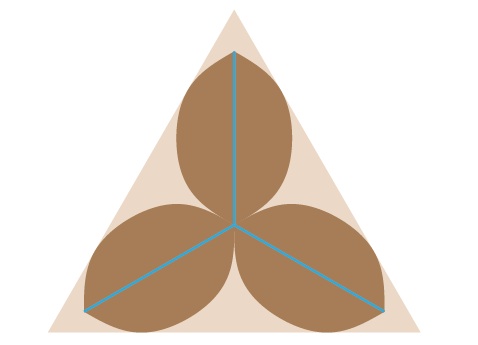
Unfortunately these four-petal wrappers cannot be packed so efficiently. However, the companion three-petal wrappers pack extremely well, and they have a number of other desirable properties:

There's plenty more. And all of it inspired by one delicious mathematical sweet.

Burkard Polster teaches mathematics at Monash and is the university's resident mathemagician, mathematical juggler, origami expert, bubble-master, shoelace charmer, and Count von Count impersonator.

Marty Ross is a mathematical nomad. His hobby is helping Barbie smash calculators and iPads with a hammer.