The maths of planet Mars

This year we’re celebrating the Maths of Planet Earth, a great and noble effort to understand and to promote the “essential role of the mathematical sciences in facing the challenges to our planet”. But what about other planets? If the Martians were similarly studying their home planet what might their mathematics be like?

Of course, imagining how Martians might think about mathematics, or anything, is definitely entering the realm of science fiction. However, it is not surprising (at least to fans of the genre) that science fiction can offer some genuine insight into mathematical fact.

One of the great early science fiction stories is A Martian Odyssey by Stanley G. Weinbaum. Written in 1934, it’s a classic of the era of pulp science fiction. The story is set in the 21st century, with astronaut Dick Jarvis trekking back to his spaceship and along the way meeting all manner of exotic Martians. There’s no attempt at deeper meaning: it is simply intended to be, and is, great fun.

However Weinbaum put some work into creating his weird creatures, and his story was concerned less with Martian actions than Martian thought. The result is a wonderful encounter of alien minds, an encounter in which simple mathematics plays a central role.

The main Martian character, and the real hero of Weinbaum’s story, is Tweel, an ostrich-like creature that Jarvis saves from a tentacled monster. Consequently Tweel and Jarvis become friends and then attempt to communicate. Tweel learns a few English words but Jarvis can make no sense of Tweel’s sounds, is simply unable to figure out how Tweel thinks. However, Jarvis gets an idea:

“After a while I gave up the language business, and tried mathematics. I scratched two plus two equals four on the ground, and demonstrated it with pebbles. Again Tweel caught the idea, and informed me that three plus three equals six.”

That gave them some form of communication, if only just: “We could exchange ideas up to a certain point, and then – blooey!” However Tweel turns out to be very inventive with the little mathematics they share.

Tweel accompanies Jarvis on his trek and they come across a rock creature: the creature repeatedly builds a pyramid about itself, breaks out and continues on to build the next, larger pyramid. Weinbaum makes no attempt to explain this fantastic creature. Tweel simply summarises with “No one-one-two. No two-two-four”.

Soon after, Jarvis begins to hallucinate and awakens to find himself entwined by a second tentacled monster. This time it is Tweel’s turn to save Jarvis, after which he explains the monster as best he can: “You one-one-two, he one-one-two.”

Finally, Jarvis and Tweel run into an army of barrel-shaped creatures, busily building mounds for no apparent reason. Tweel’s description: “One-one-two yes! Two-two-four no!” That pretty much ends the story; there’s a final confrontation with the barrel creatures, after which Jarvis is rescued by his fellow astronauts.

Weinbaum’s story is wonderfully entertaining precisely because he makes no attempt to explain his fantastic creatures. We have the vivid impression that Tweel is thinking but without any real sense of how. And Tweel is conveying something about the way the barrel creatures think but the readers are still as perplexed as Jarvis.

Obviously science fiction has no shortage of alien creatures, and if they’re not simply monsters to be fought then there is usually the issue of communication. Of course it is easiest to just have the aliens speak English, but occasionally the issue is addressed. The writer imagines, as did Weinbaum, that aliens may not just think but also think dramatically differently to we earthlings.

So the question is, how does one communicate something, anything, in the most reliable manner? Weinberg’s answer is compelling: mathematics offers the clearest, most fundamental and universal form of truth. If our alien cannot understand that 1 + 1 = 2 then it is difficult to imagine that there can be any meeting of the minds.

But then how far does it extend? Would an alien that understands 1 + 1 = 2 automatically know that 2 + 2 = 4? What about prime numbers or Pythagoras or π? It’s an interesting and instructive exercise to consider just how deep and how natural are our mathematical ideas.

In the movie Contact (based upon Carl Sagan's novel), the aliens' use of prime numbers gives the clue that their radio signals are not naturally occurring. That is very clever, even if the scriptwriter flubs the critical scene by having Jodie Foster refer to the primes as "base ten numbers".

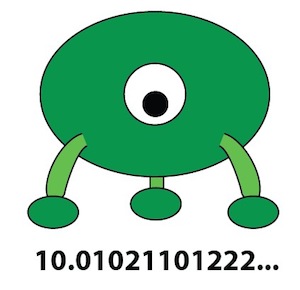
More amusing is the attempt to communicate with the aliens in Red Planet Mars by sending out the digits of π; it is perhaps reasonable to presume the Martians know of π, but sending its digits is perhaps not the most clearly thought out plan. Below we've pictured a three-legged Martian pondering his own preferred expression for π.

Of course considering how to communicate with aliens is not just the province of science fiction; there is no shortage of SETI fans pondering the problem. The Arecibo message, Earth’s most famous hello to the Universe, is intimately mathematical. The message consists of a string of 1679 0s and 1s, the number 1679 chosen because it is the product 73 x 23 of two primes. The idea is that our alien friend out there will think to arrange the numbers in a rectangle. The resulting picture then indicates fundamental facts about numbers, chemistry, we earthlings and our solar system.

Undoubtedly the most elaborate work in the attempt to communicate with aliens is LINCOS. This was an artificial language created in 1960 by Dutch mathematician Hans Freudenthal (more famous for establishing the Freudenthal Institute). Freudenthal literally begins with the whole numbers and 1 + 1 = 2, works his way through elementary mathematics, on to language and human behavior, and ends with a description of planetary motion and our solar system. It is a remarkable and hilarious achievement; Freudenthal’s 200 page message may be suitable for a curious and very patient alien but is absolutely unreadable by a human being.

So what to make of it all? How should we really try to talk to our potential alien friends? Lord knows. But mathematics seems as good an idea as any. And perhaps we can beam Stanley Weinbaum’s wonderful story: any intelligent alien should definitely appreciate that.

Free Public Lecture, Sunday September 1: Marty will give a presentation entitled The Maths of Planet Mars. Come and see Marty at the Royal Society of Victoria, 8 La Trobe Street, Melbourne, 11:30-12:30. For more information and to register, please visit the MAV website.

Burkard Polster teaches mathematics at Monash and is the university's resident mathemagician, mathematical juggler, origami expert, bubble-master, shoelace charmer, and Count von Count impersonator.

Marty Ross is a mathematical nomad. His hobby is smashing calculators with a hammer.

Copyright 2004-∞ All rights reserved.